Articles
- Page Path
- HOME > J Korean Powder Metall Inst > Volume 22(5); 2015 > Article
-
ARTICLE
- Parameter Investigation for Powder Compaction using Discrete-Finite Element Analysis
- Jinnil Choi*
-
Journal of Korean Powder Metallurgy Institute 2015;22(5):337-343.
DOI: https://doi.org/10.4150/KPMI.2015.22.5.337
Published online: September 30, 2015
Hanbat National University, Mechanical Engineering, Daejeon 34158, Korea
- *Corresponding author : : Jinnil Choi, TEL: +82-42-821-1083, FAX: +82-42-821-1083,jlchoi@hanbat.ac.kr
• Received: October 10, 2015 • Revised: October 21, 2015 • Accepted: October 20, 2015
© The Korean Powder Metallurgy Institute All rights reserved
This is an Open-Access article distributed under the terms of the Creative Commons Attribution Non-Commercial License (http://creativecommons.org/licenses/by-nc/3.0) which permits unrestricted non-commercial use, distribution, and reproduction in any medium, provided the original work is properly cited.
- 616 Views
- 6 Download
Abstract
- Powder compaction is a continually and rapidly evolving technology where it is a highly developed method of manufacturing reliable components. To understand existing mechanisms for compaction, parameter investigation is required. Experimental investigations on powder compaction process, followed by numerical modeling of compaction are presented in this paper. The experimental work explores compression characteristics of soft and hard ductile powder materials. In order to account for deformation, fracture and movement of the particles, a discrete-finite element analysis model is defined to reflect the experimental data and to enable investigations on mechanisms present at the particle level. Effects of important simulation factors and process parameters, such as particle count, time step, particle discretization, and particle size on the powder compaction procedure have been explored.
- The powder compaction process can be defined as the forming of powder material by compaction in a container into a desired single cohesive part. Powder compaction is widely used to manufacture products with various materials, such as tool and wear parts, structural parts, electrical and magnetic components, pharmaceutical tablets [1-3]. There are two main methods of modeling the powder compaction process, namely macro and micro-mechanical modeling. The macromechanical model considers powder particles as a continuum and utilizes the finite element method as the analysis tool [4-5]. Accurate input parameters are required such as geometry of tool and powders, material properties, and definition of the force applied. In micro-mechanical modelling, powder particles are considered individually and are modeled using discrete element method [6-8]. It involves interaction between particles and is designed to incorporate the contact situation for a large number of particles, where separate contact definition is not required.
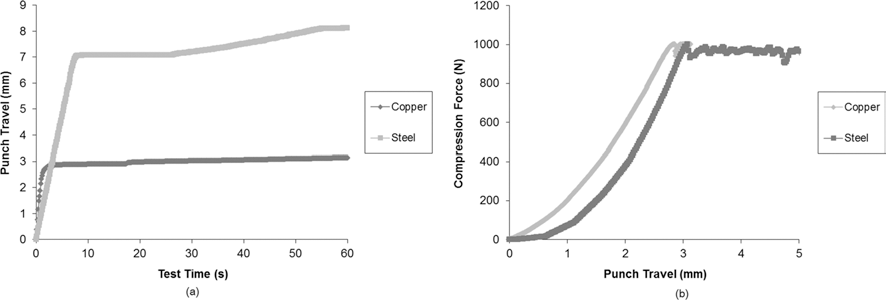
- In order to account for deformation, fracture and movement of the particles, combination of finite and discrete element method have been reported [9-12]. It follows the characteristics of a micro-mechanical model with discrete elements being divided into finite elements, shown above in Fig. 1. In this paper, in order to explore existing mechanisms in powder compaction, a discrete-finite element analysis model is defined to reflect the experimental data on compaction of powders and to enable investigations at the particle level. Effects of important simulation factors and process parameters, such as particle count, time step, particle discretization, and particle size on the powder compaction procedure have been explored.
Introduction
- Powder materials used for the experimental procedure and modeling are itemized in Table 1. To investigate plastic behavior of the material, soft and hard ductile materials were chosen and therefore a von Mises criterion was applied as one of the material properties. The model requires specification of elastic properties, the yield stress and hardening response of the material, capturing the increased resistance within each particle as deformation proceeds.
- Material properties of powders.
- Low load of 1 kN was applied using a press after 10 g of metal powder has been filled in the manufactured die for each experiment. Control stabilization for the press and consistency experiments were performed to ensure accuracy of the data.
- For modeling, particle scale compactions models have been developed to explore compaction response. Compaction in the model was achieved by downward motion through a set distance and the friction between the powder and die was assigned. These models consist of identical spherical particles in a regular matrix. A number of case studies were considered for simulation, where exploration of important simulation factors and process parameters were performed.
Experimental
Table 1.
| Powder | Young’s Modulus (GPa) | Particle Size (μm) | Yield Stress (Nmm–2) | Hardening Modulus (Nmm–2) |
|---|---|---|---|---|
|
|
||||
| Copper (OFHC Cu) | 115 | 20~30 | 240 | 440 |
| Steel (316L) | 200 | 45~70 | 50 | 440 |
- 3.1 Experimental Data
- Fig. 2 illustrates the force build up and confirms it achieves a stable level of 1 kN for the duration of the experiment. Observing the force evolution patterns, ductile powders exhibit a very sharp build up. This is most likely due to particle shape, where both steel and copper powders are spherical, and hence closely packed.
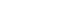
- Fig. 3(a) depicts the punch displacement curves that achieve typically a 1 kN/s loading rate. Copper compacts very quickly due to its low modulus in comparison to steel. Both powders exhibit some further punch displacement particularly for the steel powder. Fig. 3(b) shows the characteristic force against displacement data. This confirms the response similarity for the metal powders.
- 3.2 Compaction modeling 3.2.1
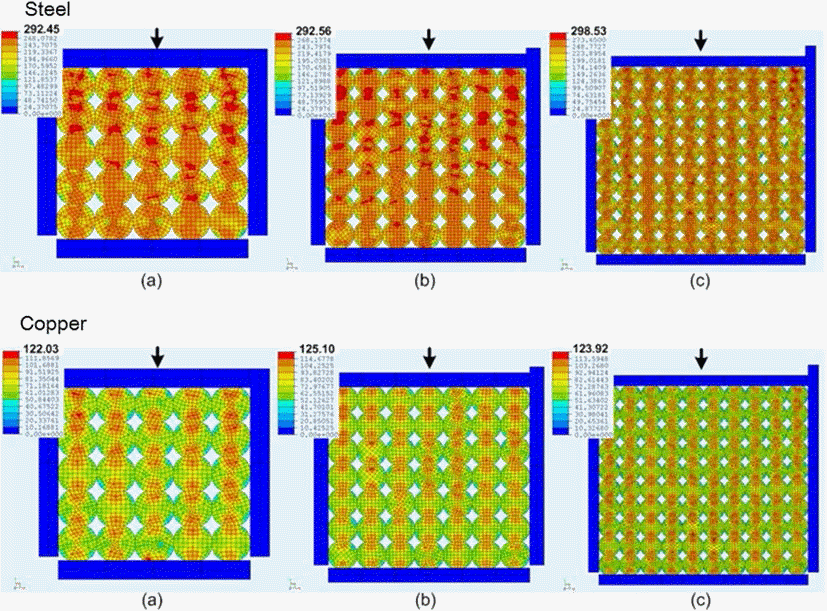
- Input loads for the compaction model was applied by downward movement of the top punch. Compaction was achieved by moving the top punch with a given displacement with the same termination time. The friction between the powder and die was assigned a coefficient value of 0.1. Fig. 4 shows the effective stress contours within each particle at notionally identical top punch displacement. Clearly, the stress values are similar for different number of particles and in comparing hard (steel) and soft (copper) materials, the difference is apparent.
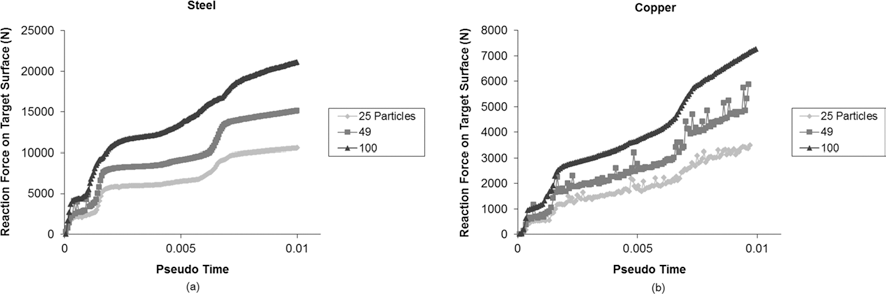
- Fig. 5 shows the evolution of force on the target surface as compaction proceeds. This also includes particle count effects for both hard and soft materials. The compaction force displays step increases for all cases, especially for the steel particles. These results were also expressed as an average load on the target surface since the dimension of the die varied for each case (Fig. 4). Since the model was two-dimensional, the force was divided by the length of the target surface. It can be seen that the general pattern was similar for different particle counts. For copper material, a more instable response could be observed, particularly for the cases of 25 and 49 particles. In general, it can be seen that a model with higher number of particles produced the smoother increase in force and thus resulting in a clearer representation of the compaction process. This was especially apparent in the case of softer material. 3.2.2
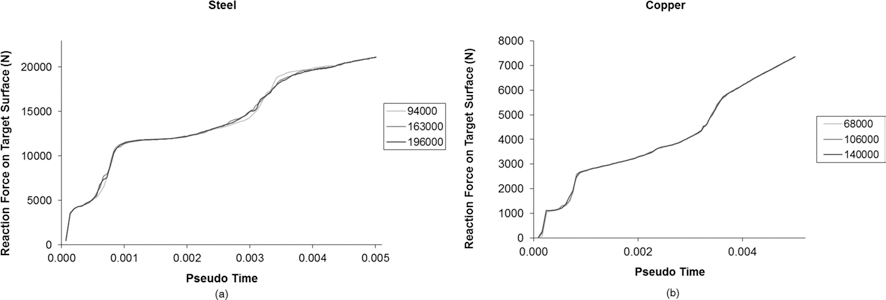
- Time step effects were explored by varying time increment with set termination time of the simulation process. For identical punch displacement and termination time, the number of divisions is increased. The critical time step value depends on material and discretization issues. Simulations were undertaken using a model comprising 100 particles with friction between the powder and tool surfaces being captured through a coefficient value of 0.1. The results from the simulations for different number of divisions are shown in Fig. 6 and little difference in effective stress was observed.
- Although it can be observed from Fig. 7 that generally the increase in number of time steps had little influence on the force value, the effect of a larger number of divisions can be observed. For the harder material, a smoother increase in force was observed. The reaction force difference for the softer material was even less. This could be due to the contact and rearrangement process of particles, which is more evident for the harder particles, while the softer particles deform plastically. 3.3.3
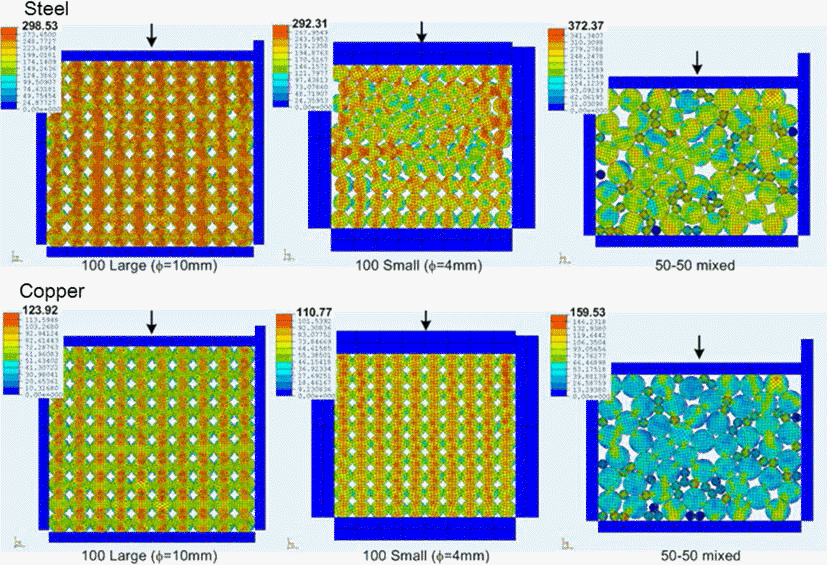
- From examining Fig. 8, the stress levels are not significantly different. Differences that do exist may arise from slight discrepancies in time at which the contours have been plotted, a consequence of data output control from the software. Each particle within the assembly was discretized identically.
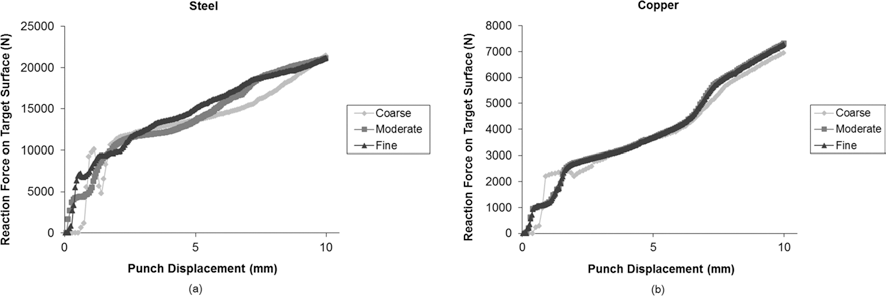
- Fig. 9 shows the reaction force evolution for which notable differences may be observed for the harder material. The force fluctuation observed for these particles diminished as the discretization became more fine. 3.3.4
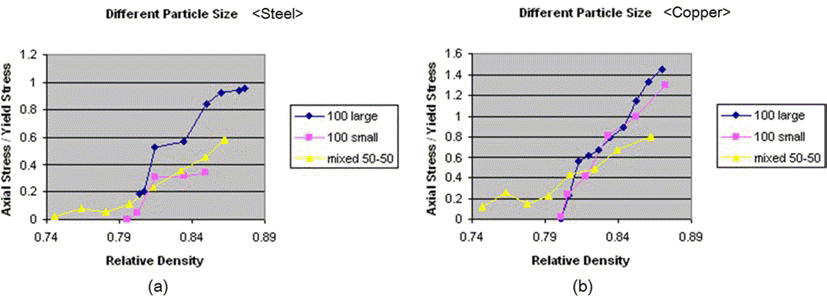
- Models with different size of particles are shown in Fig. 10. Although dimensions of the tool sets are different,constant percentage of compactions were made for identical particles in a regular matrix. For the mixed 50- 50 model, two sizes of particles were used, leading to different initial density. This can be clearly observed from Fig. 11(b), where the initial relative density of the mixture of powders was much lower than the other two models. However, the mixed model reflects a more realistic experimental environment and therefore displays a similar force evolution pattern. Variation of particle size had larger impact on harder ductile material. This seems to be because numerous rearrangement processes taking place for harder particles, while the model with softer material almost maintains the original geometry.
Results and Discussion
Fig. 3.
Graphs of (a) punch displacement against time and (b) compression force against punch displacement.

Particle count effects
Time step effects
Particle discretization effects
Fig. 8.

Schematic diagrams of effective stress with particle discretization (a) Coarse, (b) Moderate and (c) Fine.

Particle size
- Experimental investigations on powder compaction process were reported for soft and hard ductile materials. Compression force of 1 kN was applied and consistency was achieved for the duration of the experiment. Due to spherical nature of particles, similar response for the metal powders was confirmed. Steel exhibits a very sharp force build up and copper compacts very quickly due to its low modulus.
- The compaction stage was numerically modeled by use of a discrete-finite element analysis to explore compaction mechanisms. Effects of important simulation factors and process parameters, such as particle count, time step, particle discretization, and particle size on the powder compaction procedure have been explored. It is clearly seen that a model with large number of particles and time steps with fine discretization produces better results. However, since it is more time consuming to compute, optimization procedure is required. Round regular packing led to prescribing a high density, exhibiting a stiff response. Rearrangements, such as rotation and slip motion of particles had effects on the compaction, resulting in a step increase in force. Practical initial density was observed from a random packing of particles displaying a more realistic reflection of an experimental environment.
Conclusions
-
Acknowledgements
- This research was supported by the Basic Science Research Program through the National Research Foundation of Korea (NRF) funded by the Ministry of Science, ICT & Future Planning (NRF-2014R1A1A1008010).
Acknowledgements
- 1. DC Daniel, P Lindskog, H Staf and PL Larsson, J Mater. Eng. Perform. (2014) 23 –2199.
- 2. F Guner, ON Cora and H Sofuoglu, Powder Technol. (2015) 271 –238.
- 3. Z Cai, R Wang, C Peng and C Zhang, Metall Mater. Trans. B. (2015) 46 –824.
- 4. AR Khoei, Mater Des. (2002) 23 –523.
- 5. J Almanstotter, Int J. Refract. Met. Hard Mater. (2015) 50 –290.
- 6. Y He, TJ Evans, AB Yu and RY Yang, Procedia Eng. (2015) 102 –1390.
- 7. O Skrinjar and PL Larsson, Comput Mater. Sci. (2004) 31 –131.
- 8. DT Gethin and RW Lewis, Modelling Simul Mater. Sci. Eng. (2003) 11 –101.
- 9. JL Choi and DT Gethin, Modelling Simul Mater. Sci. Eng. (2009) 17 –035005.
- 10. AA Bagherzadeh Kh., A Mirghasemi and S Mohammadi, Powder Technol. (2011) 205 –15.
- 11. JP Morris, MB Rubin, GI Block and MP Bonner, Int J. Impact Eng. (2006) 33 –463.
- 12. TK Nguyen, G Combe, D Caillerie and J Desrues, Acta Geophys. (2014) 62 –1109.
REFERENCES
Figure & Data
References
Citations
Citations to this article as recorded by 

Parameter Investigation for Powder Compaction using Discrete-Finite Element Analysis
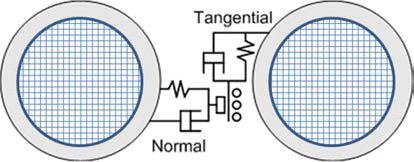
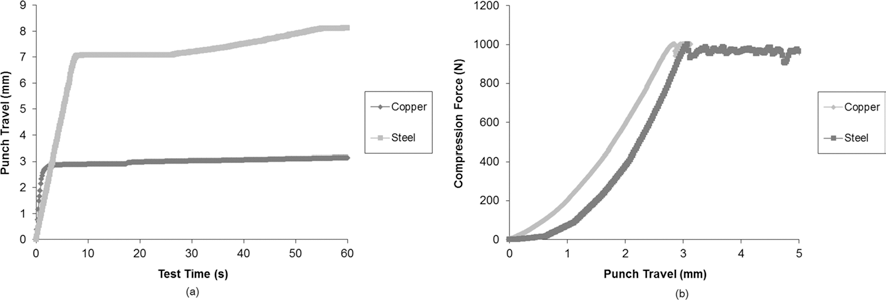
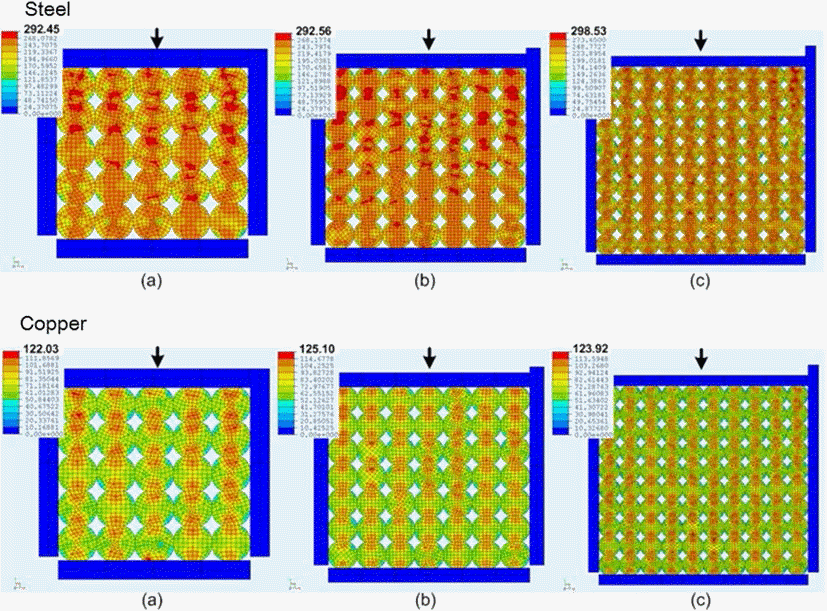



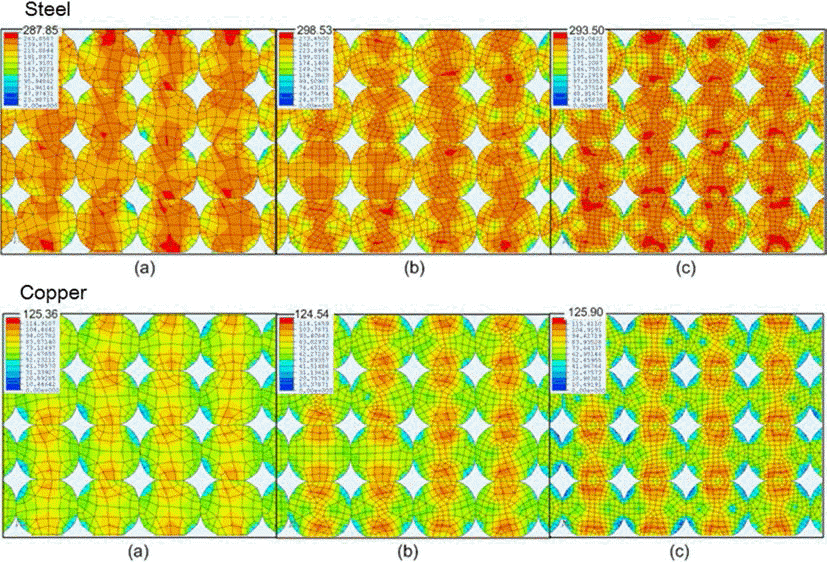
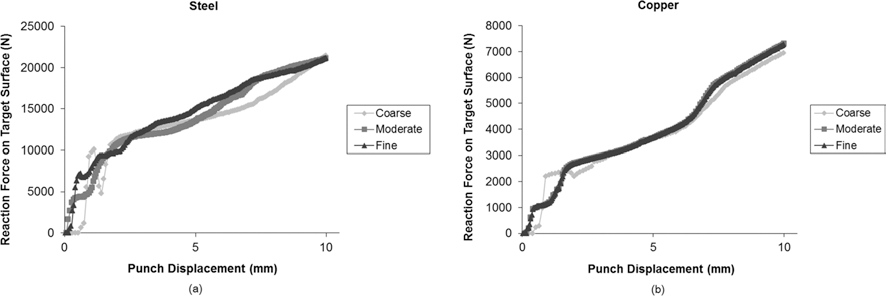

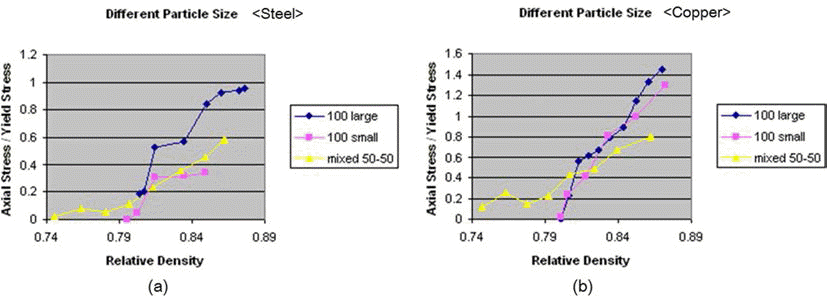
Fig. 1.
Schematic diagram of a discrete-finite element model [9].
Fig. 2.
Graphs of normal force against test time.
Fig. 3.
Graphs of (a) punch displacement against time and (b) compression force against punch displacement.
Fig. 4.
Schematic diagrams of effective stress for (a) 25 (b) 49 and (c) 100 particles.
Fig. 5.
Graphs of particle count effects with force against time for (a) Steel and (b) Copper.
Fig. 6.
Schematic diagrams of effective stress where time increment is (a) > (b) > (c).
Fig. 7.
Graphs of time step effects with (a) Steel and (b) Copper for different number of divisions.
Fig. 8.
Schematic diagrams of effective stress with particle discretization (a) Coarse, (b) Moderate and (c) Fine.
Fig. 9.
Graphs of particle discretization effects with (a) Steel and (b) Copper.
Fig. 10.
Schematic diagrams of effective stress for different particle size.
Fig. 11.
Graphs of relative density against stress ratio for different size cases with (a) Steel and (b) Copper.
Fig. 1.
Fig. 2.
Fig. 3.
Fig. 4.
Fig. 5.
Fig. 6.
Fig. 7.
Fig. 8.
Fig. 9.
Fig. 10.
Fig. 11.
Parameter Investigation for Powder Compaction using Discrete-Finite Element Analysis
| Powder | Young’s Modulus (GPa) | Particle Size (μm) | Yield Stress (Nmm–2) | Hardening Modulus (Nmm–2) |
|---|---|---|---|---|
|
|
||||
| Copper (OFHC Cu) | 115 | 20~30 | 240 | 440 |
| Steel (316L) | 200 | 45~70 | 50 | 440 |
Table 1.
Material properties of powders.
Table 1.
TOP
 kpmi
kpmi




 Cite this Article
Cite this Article